Tag Archives: teaching

Building a Probability Cannon

3 Replies

For just a moment, let's consider a staple of the second year algebra curriculum: the one-dimensional projectile motion problem.  (I used to do an awful lot of this sort of thing.)  It's not a fantastic problem---it's overdone, and often under-well---but it's representative of many of our standard modeling problems in some important ways:

  1. Every one of my students has participated in the activity we're modeling.  They've thrown, dropped, and shot things.  They've jumped and fallen and dove from various heights.  In other words, they have a passing acquaintance with gravity.
  2. Data points are relatively easy to come by.  All we need is a stopwatch and a projectile-worthy object.  If that's impractical, then there are also some great and simple---and free---simulations out there (PhET, Angry Birds), and some great and simple---and free---data collection software as well (Tracker).
  3. We only need a few data points to fix the parameters.  For a general quadratic model, we only need three data points to determine the particular solution.  Really we only need two, if we assume constant acceleration.
  4. Experiments are easy to repeat.  Drop/throw/shoot the ball again.  Run the applet again.
  5. The model conforms to a fairly nice and well-behaved family of functions.  Quadratics are continuous and differentiable and smooth, and they're generally willing to submit to whatever mathematical poking we're wont to visit upon them without getting gnarly.
  6. Theoretical predictions are readily checked.  Want to know, for instance, when our projectile will hit the ground?  Find the sensible zero of the function (it's pretty easy to sanity check its reasonableness---see #1 above).  Look at a table of values and step through the motion second-by-second (use a smaller delta t for an even better sense of what's going on).  Click RUN on your simulation, and wait until it stops (self-explanatory).  And, if you're completely dedicated, build yourself a cannon and put your money where your mouth is.

Of course I've chosen to introduce this discussion with the example of projectile motion, but there are plenty of other candidates: length/area/volume, exponential growth and decay, linear speed and distance.  Almost without exception (in the algebra classroom), we model phenomena that satisfy the six conditions listed above.

Almost.  Because then we run into probability, and probability isn't so tame.  I'll grant that #1 still holds (though I'm not entirely convinced it holds in the same sense), but the other five conditions go out the window.

Data points are NOT easy to come by.

I can already hear you protesting.  "Flip a coin...that's a data point!"  Well, yes.  Sort of.  But in the realm of probability, individual data points are ambiguous.  The ordered pair (3rd flip, heads) is very different from (3 seconds, 12 meters).  They're both measurements, but the first one has much, much higher entropy.  Interpretation becomes problematic.  Here's another example: My meteorologist's incredibly sophisticated model (dart board?) made the following prediction yesterday: P(rain) = 0.6.  In other words, the event "rain" was more likely than the event "not rain."  It did not rain yesterday.  How am I to understand this un-rain?  Was the model right?  If so, then I'm not terribly surprised it didn't rain.  Was the model wrong?  If so, then I'm not terribly surprised it didn't rain.  In what sense have I collected "data?"

And what if I'm interested in a compound event?  What if I want to know not just the result of a lone flip, but P(exactly 352 heads in 1000 flips)?  Now a single data point suddenly consists of 1000 trials.  So it turns out data points have the potential to be rather difficult to come by, which brings us to...

We need an awful lot of data points.

I'm not talking about our 1000-flip trials here, which was just a result of my arbitrary choice of one particular problem.  I mean that, no matter what our trials consist of, we need to do a whole bunch of them in order to build a reliable model.  Two measurements in my projectile problem determine a unique curve and, in effect, answer any question I might want to ask.  Two measurements in a probabilistic setting tell me just about nothing.

Consider this historical problem born, like many probability problems, from gambling.  On each turn, a player rolls three dice and wins or loses money based on the sum (fill in your own details if you want; they're not so important for our purposes here).  As savvy and degenerate gamblers, we'd like to know which sums are more or less likely.  We have some nascent theoretical ideas, but we'd like to test one in particular.  Is the probability of rolling a sum of 9 equal to the probability of rolling a sum of 10?  It seems it should be: after all, there are six ways to roll a 9 ({6,2,1},{5,3,1},{5,2,2},{4,4,1},{4,3,2},{3,3,3}), and six ways to roll a 10 ({6,3,1},{6,2,2},{5,4,1},{5,3,2},{4,4,2},{4,3,3})*.  Done, right?

It turns out this isn't quite accurate.  For instance, the combination {6,2,1} treats all of the 3! = 6 permutations of those numbers as one event, which is bad mojo.  If you go through all 216 possibilities, you'll find that there are actually 27 ways to roll a 10, and only 25 ways to roll a 9, so the probabilities are in fact unequal.  Okay, no biggie, our experiment will certainly show this bias, right?  Well, it will, but if we want to be 95% experimentally certain that 10 is more likely, then we'll have to run through about 7,600 trials!  (For a derivation of this number---and a generally more expansive account---see Michael Lugo's blog post.)  In other words, the Law of Large Numbers is certainly our friend in determining probabilities experimentally, but it requires, you know, large numbers.

*If you've ever taught probability, you know that this type of dice-sense is rampant.  Students consistently collapse distinct events based on superficial equivalence rather than true frequency.  Ask a room of high school students this question: "You flip a coin twice.  What's the probability of getting exactly one head?"  A significant number will say 1/3.  After all, there are three possibilities: no heads, one head, two heads.  Relatively few will immediately notice, without guidance, that "one head" is twice as likely as the other two outcomes.

Experiments are NOT easy to repeat.

I've already covered some of the practical issues here in terms of needing a lot of data points.  But beyond all that, there are also philosophical difficulties.  Normally, in science, when we talk about repeating experiments, we tend to use the word "reproduce."  Because that's exactly what we expect/are hoping for, right?  I conduct an experiment.  I get a result.  I (or someone else) conduct the experiment again.  I (they) get roughly the same result.  Depending on how we define our probability experiment, that might not be the case.  I flip a coin 10 times and count 3 heads.  You flip a coin 10 times and count 6 heads.  Experimental results that differ by 100% are not generally awesome in science.  In probability, they are the norm.

As an interesting, though somewhat tangential observation, note that there is another strange philosophical issue at play here.  Not only can events be difficult to repeat, but sometimes they are fundamentally unrepeatable.  Go back to my meteorologist's prediction for a moment.  How do I repeat the experiment of "live through yesterday and see whether it rains?"  And what does a 60% chance of rain even mean?  To a high school student (teacher) who deals almost exclusively in frequentist interpretations of probability, it means something like, "If we could experience yesterday one million times, about 600,000 of those experiences would include rain."  Which sounds borderline crazy.  And the Bayesian degree-of-belief interpretation isn't much more comforting: "I believe, with 60% intensity, that it will rain today."  How can we justify that level of belief without being able to test its reliability by being repeatedly correct?  Discuss.

Probability distributions can be unwieldy.

Discrete distributions are conceptually easy, but cumbersome.  Continuous distributions are beautiful for modeling, but practically impossible for prior-to-calculus students (not just pre-calculus ones).  Even with the ubiquitous normal distribution, there is an awful lot of hand-waving going on in my classroom.  Distributions can make polynomials look like first-grade stuff.

Theoretical predictions aren't so easily checked.

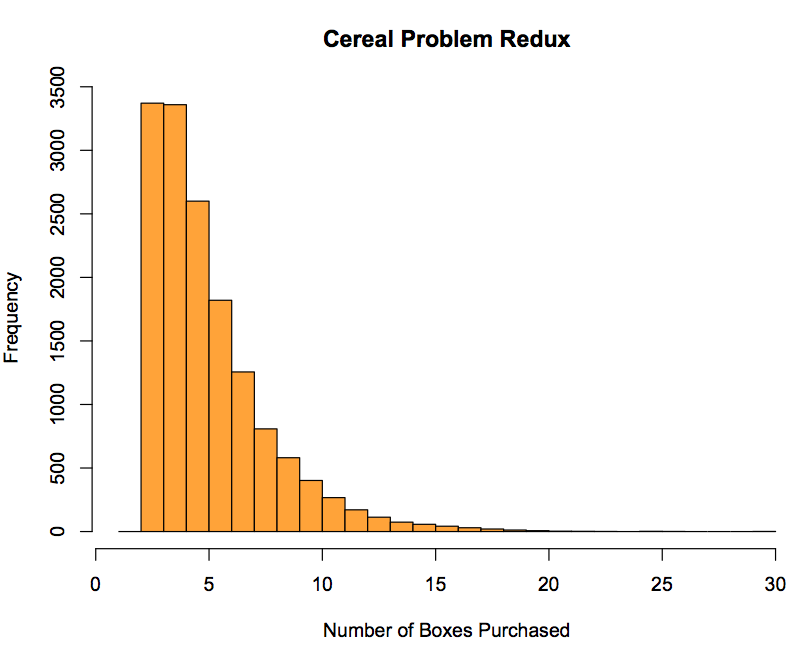
My theoretical calculations for the cereal box problem tell me that, on average, I expect to buy between 5 and 6 boxes to collect all the prizes.  But sometimes when I actually run through the experiment, it takes me northward of 20 boxes!  This is a teacher's nightmare.  We've done everything right, and then suddenly our results are off by a factor of 4.  Have we confirmed our theory?  Have we busted it?  Neither?  Blurg.  So what are we to do?

We are to build a probability cannon!

With projectile motion problems, building a cannon is nice.  It's cool.  We get to launch things, which is awesome.  With probability, I submit that it's a necessity.  We need to generate data: it's the raw material from which conjecture is built, and the touchstone by which theory is tested.  We need to (metaphorically) shoot some stuff and see where it lands.  We need...simulations!

If your model converges quickly, then hand out some dice/coins/spinners.  If it doesn't, teach your students how to use their calculators for something besides screwing up order of operations.  Better yet, teach them how to tell a computer to do something instead of just watching/listening to it.  (Python is free.  If you own a Mac, you already have it.)  Impress them with your wizardry by programming, right in front of their eyes, and with only a few lines of code, dice/coins/spinners that can be rolled/flipped/spun millions of times with the push of a button.  Create your own freaking distributions with lovely, computer-generated histograms from your millions of trials.  Make theories.  Test theories.  Experience anomalous results.  See that they are anomalous.  Bend the LLN to your will.

Exempli Gratia

NCTM was kind enough to tweet the following problem today, as I was in the middle of writing this post:

Okay, maybe the probability is just 1/2.  I mean, any argument I make for Kim must be symmetrically true for Kyle, right?  But wait, it says "greater than" and not "greater than or equal to," so maybe that changes things.  Kim's number will be different from Kyle's most of the time, and it will be greater half of the times it's different, so...slightly less than 1/2?  Or maybe I should break it down into mutually exclusive cases of {Kim rolls 1, Kim rolls 2, ... , Kim rolls 6}.  You know what, let's build a cannon.  Here it is, in Mathematica:

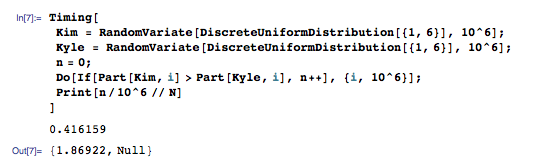Okay, so it looks like my second conjecture is right; the probability is a little less than 1/2.  Blammo!  And it only took (after a few seconds of typing the code) 1.87 seconds to do a million trials.  Double blammo!  But how much less than 1/2?  Emboldened by my cannon results, I can turn back to the theory.  Now, if Kyle rolls a one, Kim will roll a not-one with probability 5/6.  Ditto two, three, four, five, and six.  So Kim's number is different from Kyle's 5/6 of the time.  And---back to my symmetry argument---there should be no reason for us to believe one or the other person will roll a bigger number, so Kim's number is larger 1/2 of 5/6 of the time, which is 5/12 of the time.  Does that work?  Well, since 5/12 ≈ 0.4167, which is convincingly close to 0.416159, I should say that it does.  Triple blammo and checkmate!

But we don't have to stop there.  What if I remove the condition that Kim's number is strictly greater?  What's the probability her number is greater than or equal to Kyle's?  Now my original appeal to symmetry doesn't require any qualification.  The probability ought simply be 1/2.  So...

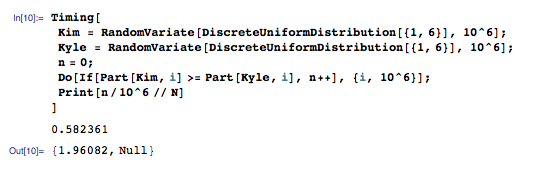What what?  Why is the probability greater than 1/2 now?  Oh, right.  Kim's roll will be equal to Kyle's 1/6 of the time, and we already know it's strictly greater than Kyle's 5/12 of the time.  Since those two outcomes are mutually exclusive, we can just add the probabilities, and 1/6 + 5/12 = 7/12, which is about (yup yup) 0.583.  Not too shabby.

What if we add another person into the mix?  We'll let Kevin join in the fun, too.  What's the probability that Kim's number will be greater than both Kyle's and Kevin's?

It looks like the probability of Kim's number being greater than both of her friends' might just be about 1/4.  Why?  I leave it as an exercise to the reader.

That tweet-sized problem easily becomes an entire lesson with the help of a relatively simple probability cannon.  If that's not an argument for introducing them into your classroom, I don't know what is.

Ready.  Aim.  Fire!

Thanks to Christopher Danielson for sparking this whole discussion.

A Tale of Two Numbers

7 Replies

A few months ago, we had just finished talking about polynomials and were moving into matrices.  Because a lot of matrix concepts have analogs in the real numbers, we kicked things off with a review of some real number topics.  Specifically, I wanted to talk about solving linear equations using multiplicative inverses as a preview of determinants and using inverse matrices for solving linear systems.  For instance:

$latex begin{array}{ll}

2x=8 & AX=B \

2^{-1}2x = 2^{-1}8 & A^{-1}AX = A^{-1}B \

1x = frac{1}{2}8 & IX = A^{-1}B \

x=4 & X = A^{-1}B

end{array}&s=2$

As an aside, I threw out this series of equations in the hopes of (a) foreshadowing singular matrices, and (b) offering a justification for the lifelong prohibition against dividing by zero:

$latex begin{array}{l}

0x=1 \

0^{-1}0x = 0^{-1}1 \

1x = frac{1}{0}1 \

x = frac{1}{0}

end{array}&s=2$

I thought this was just so beautiful.  Why can't we divide by zero?  Because zero doesn't have a multiplicative inverse.  There is no solution to 0x = 1, so 0-1 must not exist!  Q.E.D.

As it turns out, Q.E.NOT.  One of my students said, "Why can't we just invent the inverse of zero?  Like we did with i?"

Again, we had just finished our discussion of polynomials, during which we had conjured the square root of -1 seemingly out of the clear blue sky.  They wanted to do the same thing with 1/0.  What an insightful and beautiful idea!  Consider the following stories, from my students' perspectives:

  1. When we're trying to solve quadratic equations, we might happen to run into something like x2 = -1.  Now of course there is no real number whose square is -1, so for convenience let's just name this creature i (the square root of -1), and put it to good use immediately.
  2. When we're trying to solve linear equations, we might happen to run into something like 0x = 1.  Now of course there is no real number that, when multiplied by 0, yields 1, so for convenience let's just name this creature j (the multiplicative inverse of 0), and put it to good use immediately.

Why are we allowed to do the first thing, but not the second?  Why do we spend a whole chapter talking about the first thing, and an entire lifetime in contortions to avoid the second?  Both creatures were created, more or less on the spot, to patch up shortcomings in the real numbers.  What's the difference?

And this is the tricky part: how do I explain it within the confines of a high school algebra class?  Well, I can tell you what I tried to do...

Let's suppose that j is a legitimate mathematical entity in good standing with its peers, just like i.  Since we've defined j as the number that makes 0j = 1 true, it follows that 0 = 1/j.  Consider the following facts:

$latex begin{array}{l}

2 cdot 0 = 0 \

2frac{1}{j} = frac{1}{j} \

frac{2}{j} = frac{1}{j} \

2 = 1

end{array}&s=2$

In other words, I can pretty quickly show why j allows us to prove nonsensical results that lead to the dissolution of mathematics and perhaps the universe in general.  After all, if I'm allowed to prove that 2 = 1, then we can pretty much call the whole thing off.  What I can't show, at least with my current pedagogical knowledge, is why i doesn't lead to similar contradictions.

Therein lies the broad problem with proof.  It's difficult.  If there are low-hanging fruit on the counterexample tree, then I can falsify bad ideas right before my students' very eyes.  But if there are no counterexamples, then it becomes incredibly tough.  It's easy to show a contradiction, much harder to show an absence of contradiction.  I can certainly take my kids through confirming examples of why i is helpful and useful.  But in my 50 min/day with them, there's just no way I can organize a tour through the whole scope and beauty of complex numbers.  Let's be serious, there's no way that I can even individually appreciate their scope and beauty.

The complex numbers aren't just a set, or a group.  They're not even just a field.  They form an algebra (so do matrices, which brings a nice symmetry to this discussion), and algebras are strange and mysterious beings indeed.  I could spend the rest of my life learning why i leads to a rich and self-consistent system, so how am I supposed to give a satisfactory explanation?

Take it on faith, kids.  Good enough?

Update 3/20/12: My friend, Frank Romascavage, who is currently a graduate student in math at Bryn Mawr College (right down the road from my alma mater Villanova), pointed out the following on Facebook:

"We need to escape integral domains first so that we can have zero divisors!  Zero divisors give a quasi-invertibility condition (with respect to multiplication) on 0.  They aren't really true inverses, but they are somewhat close!  In $latex Z_{6}$ we have two zero divisors, 3 and 2, because 3 times 2 (as well as 2 times 3) in $latex Z_{6}$ is 0."

In many important ways, an integral domain is a generalization of the integers, which is why they behave very much the same.  An integral domain is just a commutative ring (usually assumed to have a unity), with no zero divisors.  If there are two members of a ring, say a and b, then they are said to be zero divisors if ab = 0.  In other words, to "escape integral domains," is to move into a ring where the Zero Product Property no longer holds.  This means that, in non-integral domains, we can almost, sort of, a little bit, divide by zero.  Zero doesn't really have a true inverse, but it's close.  Frank's example is the numbers 2 and 3 in the ring of integers modulo 6, since 3 x 2 = 0 (mod 6).  In fact, the ring of integers modulo n fails to be an integral domain in general, unless n is prime.  CTL

0!rganized Emptiness

4 Replies

On the back of the fundamental counting principle, my class has just established the fact that we can use n! to count the number of possible arrangements of n unique objects.  This is fantastic, but we don't always want to arrange all of the n things available to us, which is okay.  We've also been introduced to the permutation function, which has the very nice property of counting ordered arrangements of r-sized subsets of our n objects.  Handy indeed.

Today we made an interesting observation: we now have not one, but two ways to count arrangements of, let's say, 7 objects.

  1. We can fall back on our old friend, the factorial, and compute 7!
  2. We can use our new friend, the permutation function, and compute $latex bf{_7P_7}$

Since both expressions count the same thing, they ought to be equal, but then we run into this interesting tidbit when we evaluate (2):

$latex _7P_7 = frac{7!}{(7-7)!} = frac{7!}{0!}&s=2$,

which seems to imply that 0! = 1.  To say this is counterintuitive for my kids would be a severe understatement.  And in this moment of philosophical crisis, when the book might present itself as a palliative ally, students are instead met with this:

To prevent inconsistency?  How in the world are kids supposed to trust a mathematical resource that paints itself into a corner, only tacitly admits such, and then drops a bomb of a deus ex machina in order to save face?  I haven't been so angry since the ending of Lord of the Flies.  Especially when this problem appears two pages later:

Okay, 8!.  So how many ways can I arrange my bookshelf with a zero-volume reference set?  One: I can arrange an empty shelf in exactly one way.  And, since we already know that n! counts the ways I can arrange n objects, it follows naturally that this 1 way of arranging 0 things must also be represented by 0!.

There are a lot of good proofs/justifications available for the willing Googler, but this one, to me, seems like the most natural and straightforward for a high school classroom.  At a bare minimum, it's much, much better than, "Because I need it to be true for my own convenience."

Only a math textbook could take something so lovely and make it seem dirty.

Cereal Boxes Redux

8 Replies

In my last post, my students were wrestling with a question about cereal prizes.  Namely, if there is one of three (uniformly distributed) prizes in every box, what's the probability that buying three boxes will result in my ending up with all three different prizes?  Not so great, turns out.  It's only 2/9.  Of course this raises another natural question: How many stupid freaking boxes do I have to buy in order to get all three prizes?

There's no answer, really.  No number of boxes will mathematically guarantee my success.  Just as I can theoretically flip a coin for as long as I'd like without ever getting tails, it's within the realm of possibility that no number of purchases will garner me all three prizes.  But, just like the coin, students get the sense that it's extremely unlikely that you'd buy lots and lots of boxes without getting at least one of each prize.  And they're right.  So let's tweak the question a little: How many boxes do I have to buy on average in order to get all three prizes?  That's more doable, at least experimentally.

I have three sections of Advanced Algebra with 25 - 30 students apiece.  I gave them all dice to simulate purchases and turned my classroom---for about ten minutes at least---into a mathematical sweatshop churning out Monte Carlo shopping sprees.  The average numbers of purchases needed to acquire all prizes were 5.12, 5.00, and 5.42.  How good are those estimates?

Simulating cereal purchases with dice

Here's my own simulation of 15,000 trials, generated in Python and plotted in R:

I ended up with a mean of 5.498 purchases, which is impressively close to the theoretical expected value of 5.5.  So our little experiment wasn't too bad, especially since I'm positive there was a fair amount of miscounting, and precisely one die that's still MIA from excessively enthusiastic randomization.

And now here's where I'm stuck.  I can show my kids the simulation results.  They have faith---even though we haven't formally talked about it yet---in the Law of Large Numbers, and this will thoroughly convince them the answer is about 5.5.  I can even tell them that the theoretical expected value is exactly 5.5.  I can even have them articulate that it will take them precisely one box to get the first new toy, and three boxes, on average, to get the last new toy (since the probability of getting it is 1/3, they feel in their bones that they should have to buy an average of 3 boxes to get it).  But I feel like we're still nowhere near justifying that the expected number of boxes for the second toy is 3/2.

For starters, a fair number of kids are still struggling with the idea that the expected value of a random variable doesn't have to be a value that the variable can actually attain.  I'm also not sure how to get at this next bit.  The absolute certainty of getting a new prize in the first box is self-evident.  The idea that, with a probability of success of 1/3, it ought "normally" to take 3 tries to succeed is intuitive.  But those just aren't enough data points to lead to the general conjecture (and truth) that, if the probability of success for a Bernoulli trial is p, then the expected number of trials to succeed is 1/p.  And that's exactly the fact we need to prove the theoretical solution.  Really, that's what we need basically to solve the problem completely for any number of prizes.  After that, it's straightforward:

The probability of getting the first new prize is n/n.  The probability of getting the second new prize is (n-1)/n ... all the way down until we get the last new prize with probability 1/n.  The expected numbers of boxes we need to get all those prizes are just the reciprocals of the probabilities, so we can add them all together...

If X is the number of boxes needed to get all n prizes, then

$latex E(X) = frac{n}{n} + frac{n}{n-1} + cdots + frac{n}{1} = n(frac{1}{n} + frac{1}{n-1} + cdots + frac{1}{1}) = n cdot H_n&s=2$

where Hn is the nth harmonic number.  Boom.

Oh, but yeah, I'm stuck.

Pruning Tree Diagrams

2 Replies

A few days ago we opened up with some group work surrounding the following problem.  I gave no guidance other than, "One representative will share your solution with the class."

My favorite cereal has just announced that it's going to start including prizes in the box.  There is one of three different prizes in every package.  My mom, being cheap and largely unwilling to purchase the kind of cereal that has prizes in it, has agreed to buy me exactly three boxes.  What is the probability that, at the end of opening the three boxes, I will have collected all three different prizes?

It's a very JV, training-wheels version of the coupon collector's problem, but it's nice for a couple of reasons:

  1. The actual coupon collector's problem is several years out of reach, but it's a goody, so why not introduce the basics of it?
  2. There is a meaningful conversation to be had about independence.  (Does drawing a prize from Box 1 change the probabilities for Box 2?  Truly?  Appreciably?  Is it okay to assume, for simplicity, that it doesn't?  How many prizes need to be out there in the world for us to feel comfortable treating this thing as if it were a drawing with replacement?  If everybody else is buying up cereal---and prizes---uniformly, does that bring things closer to true independence?  farther away?)
  3. There are enough intuitive wrong answers to require some deeper discussion: e.g, 1/3 (Since all the probabilities along the way are 1/3, shouldn't the final probability of success also be 1/3?), 1/27 (There are three chances of 1/3 each, so I multiplied them together.), and 1/9 (There are three shots at three prizes, so nine outcomes, and I want the one where I get all different toys.)  The correct answer, by the by, is 6/27 or 2/9 (try it out).

Many groups jumped right into working with the raw numbers (see wrong answers above).  A few tried, with varying levels of success, to list all the outcomes individually (interestingly, a lot of these groups correctly counted 27 possibilities, but then woefully miscounted the number of successes...hmmm).  A small but determined handful of groups used tree diagrams to help them reason about outcomes sequentially.

This business of using tree diagrams was pleasantly surprising.  We hadn't yet introduced them in class, and I hadn't made any suggestions whatsoever about how to tackle the problem, so I thought it was nice to see a spark of recollection.  That said, it's not terribly surprising; presumably these kids have used them before.  But I did run across one student, Z, who interpreted his tree diagram in novel way---to me at least.

Most students, when looking at a tree diagram, hunt for paths that meet the criteria for success.  Here's a path where I get Prize 1, then Prize 2, then Prize 3.  Here's another where I get Prize 1, then Prize 3, then Prize 2...  The algorithm goes something like, follow a path event-by-event and, if you ultimately arrive at the compound event of interest, tally up a success.  Repeat until you're out of paths.  That is, most students see each path as an stand-alone entity to be checked, and then either counted or ignored.

What Z did was different in three important ways.  First of all, he found his solutions via subtraction rather than addition.  Second, he attacked the problem in a very visual---almost geometric---way.  And third, he didn't treat each path separately; rather, Z searched for equivalence classes of paths within the overall tree.

Z's (paraphrased) explanation goes as follows:

First I erased all of the straight paths, because they mean I get the same prize in every box.  Then I erased all of the paths that were almost straight, but had one segment that was crooked, which means I get two of the same prize.  And then I was left with the paths that were the most crooked, which means I get a different prize each time.

Looking at his diagram, I noticed that Z hadn't even labeled the segments; he simply drew the three stages, with three possibilities at each node, and then deleted everything that wasn't maximally crooked.  How awesome is that?  In fact, taking this tack made it really easy for him to answer more complicated followup questions.  Since he'd already considered the other cases, he could readily figure out the probability of getting three of the same prize (the 3 branches he pruned first), or getting only two different prizes (the next 18 trimmings).  He could even quickly recognize the probability of getting the same prize twice in a row, followed by a different one (the 6 branches he trimmed that went off in one direction, followed by a straight-crooked pattern).

[youtube=http://www.youtube.com/watch?v=jfJb_afqA0M]

Of course this method isn't particularly efficient.  He had to cut away 21 paths to get down to 6.  For n prizes and boxes, you end up pruning nn --- n! branches.  Since nn grows much, much faster, than n!, Z's algorithm becomes prohibitively tedious in a hurry.  If there are 5 prizes and 5 boxes, that's already 3005 branches that need to be lopped off.  So yes, it's inefficient, but then again so are tree diagrams.  Without more sophisticated tools under his belt, that's not too shabby.  What the algorithm lacks in computational efficiency, it makes up for in conceptual thoughtfulness.  I'll take it that tradeoff any day of the week.

Smashmouth Mathematics

5 Replies

If it were physically possible to fold a piece of paper in half 50 times (it's not), how thick would the resulting origami sculpture be?  Quick!  No fair calculating.  What does your gut say?

If you have absolutely no idea, I'll tell you that a standard piece of printer paper, folded six times by high a school student with very little concern for symmetry or crease definition, has an average thickness somewhere between six and eight millimeters.  How much will that increase over the next 44 folds?  Any ideas?

Continue reading →

Computational Crisis

3 Replies

Let's be clear: American software engineering is in crisis.  Thirty years ago our computer programs were the best in the world; now they routinely lag behind those from South Korea, Finland, China, and even...*gulp*...Canada.  In fact, a 2009 assessment found that U.S. reading software ranked 17th among the 34 OECD countries, math software a dismal 25th.  In the absence of radical reform, our code will cease to be competitive in an increasingly global economy, and we risk losing our preeminent place on the world stage.

In addition to poor test scores, American software has been suffering from increased feelings of alienation and disengagement.  In a survey from last year, 61% of programs said that they "strongly disliked" or "hated" compiling, and more than half said they would rather digitize Wuthering Heights than debug.  There's no doubt the situation is dire.

But there is a solution.

Continue reading →

Choosy

4 Replies

Something deeply unsettling is afoot in the land of math education when I'm teaching the same backwards thing in the same backwards way it was presented to me as a high school kid.  To wit, combinations.

Here is the current state of the art, according to the big boys of Advanced Algebra publishing:

Fundamental Counting Principle ====> Permutations ====> Combinations ====> Pascal's Triangle ====> Binomial Theorem  ====> Celebration

I submit that, when we do it this way, we're double-charging our students for their attention.  We bog them down in unnecessary algebraic trifling, and we go out of our way to delay the payoff for just as long as possible.  It's bad marketing, and it's bad teaching.  And we don't exactly get away scot-free in all this.  I know I'm in for a rough couple of weeks any time I have to close my opening lesson with, "Trust me."

Continue reading →

The Dead Puppy Theorem

3 Replies

So for the past few months I've been telling my kids that, every time they write $latex (x+y)^2 = x^2+y^2$, they kill a puppy.  In fact, I will hereafter refer to that equation as the Dead Puppy Theorem, or DPT.  Since its discovery in early September, my students' usage of the DPT has accounted for more canine deaths than heartworms.

Continue reading →

noe.2.thynges

2 Replies

Thanks to the book Group Theory in the Bedroom, by Brian Hayes, I finally found someone to blame for my geometry students' daily growing hatred of mathematical language.  His name is Robert Recorde.  For those of you who, like pre-this-week me, have never heard of Robert Recorde, don't worry: you've seen his handiwork.  Recorde was a 16th-century Welsh doctor, mathematician, and author of Whetstone of Witte (1557), the book in which the modern equals sign first appears.

Continue reading →